BJT Amplifiers – Small Signal Analysis

Description

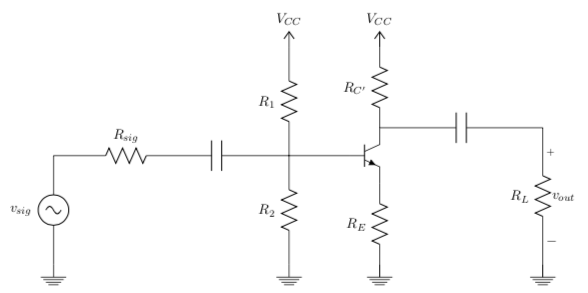
Excerpt from the Common Emitter section of document (depicted in main image of this resource):

The first figure of merit we are asked to determine is \(R_{in}\).

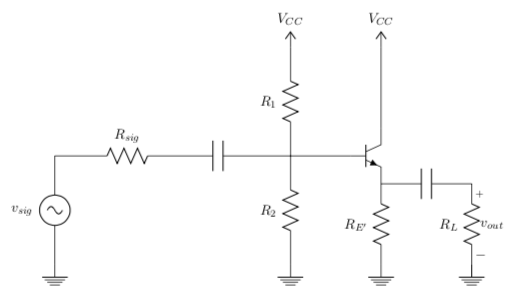
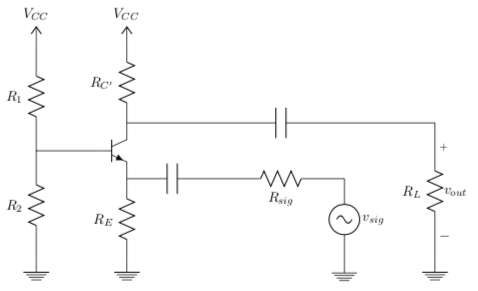
We know from Figure 5 that \(R_{in}\) represents the entire amplifier to the right of \(R_{sig}\). We also know that \(R_{in} = \frac{v_{in}}{i_{in}}\). We can determine \(v_{in}\) and \(i_{in}\) from the circuit in Figure 10.

Writing a nodal equation for \(i_{in}\) yields:

\begin{align}
i_{in} &= i_{B} + i_{b} \nonumber \\
\textit{where } i_{B} &= \frac{v_{in}}{R_{B}} \nonumber \\
i_{in} &= \frac{v_{in}}{R_{B}} + i_{b}\ \ \ \ \ (2)
\end{align}

Writing a mesh equation for \(v_{in}\) yields:

\begin{equation}
v_{in} = i_{b}r_{\pi} + i_{e}R_{E}\ \ \ \ \ (3)
\end{equation}

Let’s continue to develop \(v_{in}\) by obtaining it in terms of \(i_{b}\) since Equation (2) is in terms of \(i_{b}\). We can accomplish this by writing a nodal equation for \(i_{e}\), where:

\begin{align}
i_{e} &= i_{b} + \beta{i_{b}} \nonumber \\
&= (\beta+1)i_{b}\ \ \ \ \ (4)
\end{align}

Subbing \(i_{e}\) in Equation (4) for \(i_{e}\) in Equation (3) yields:

\begin{align}
v_{in} &= i_{b}r_{\pi} + i_{b}(\beta+1)R_{E} \nonumber \\
&= [r_{\pi} + (\beta+1)R_{E}]i_{b}\ \ \ \ \ (5)
\end{align}

Solving for \(i_{b}\) in Equation (5) produces:

\begin{equation}
i_{b} = \frac{v_{in}}{r_{\pi} + (\beta+1)R_{E}}\ \ \ \ \ (6)
\end{equation}

Substituting Equation (6) into Equation (2) for \(i_{b}\) yields:

\begin{align}
i_{in} &= \frac{v_{in}}{R_{B}} + \frac{v_{in}}{r_{\pi} + (\beta+1)R_{E}} \nonumber \\
&= \left[\frac{1}{R_{B}} + \frac{1}{r_{\pi} + (\beta+1)R_{E}}\right]v_{in}\ \ \ \ \ (7)
\end{align}

Solving for \(\frac{i_{in}}{v_{in}}\) in Equation (7) yields:

\begin{equation}
\frac{i_{in}}{v_{in}} = \frac{1}{R_{in}} = \frac{1}{R_{B}} + \frac{1}{r_{\pi} + (\beta+1)R_{E}}\ \ \ \ \ (8)
\end{equation}

From circuit theory, we know that:

\begin{equation*}
\frac{1}{R_{Parallel}} = \frac{1}{R_{x}} + \frac{1}{R_{y}}
\end{equation*}

With this knowledge we obtain \(R_{in}\) from Equation (8) as:

\( \newcommand{\parallelsum}{\mathbin{\!/\mkern-5mu//\!}} \)
\begin{equation}
\boxed{R_{in} = R_{B}\parallelsum[r_{\pi} + (\beta+1)R_{E}]}\ \ \ \ \ (9)
\end{equation}

The next figure of merit we are asked to find is the Open Circuit Voltage Gain, \(A_{v_o}\).

We can determine \(v_{out}\) by inspecting the right side of the circuit in Figure 10.

\begin{equation}
v_{out} = -\beta{i_{b}}R_{C} \ \ \ \ \ (10)
\end{equation}

Taking Equation 10 and dividing it by Equation 5 gives us:

\begin{align}
\frac{v_{out}}{v_{in}} &= \frac{-\beta{i_b}R_{C}}{[r_{\pi} + (\beta+1)R_{E}]i_{b}} \nonumber \\
&= \boxed{\frac{-\beta R_{C}}{r_{\pi} + (\beta+1)R_{E}} = A_{v_o}}\ \ \ \ \ (11)
\end{align}

We were also asked to find the Overall Circuit Voltage Gain, \(\frac{v_{out}}{v_{sig}}\).

We can do this by substituting \(v_{in}\) within Equation (1) for \(v_{in}\) within Equation (11).

\begin{align}
\frac{v_{out}}{\frac{R_{in}}{R_{in}+R_{sig}}v_{sig}} &= \frac{-\beta R_{C}}{r_{\pi} + (\beta+1)R_{E}} \nonumber \\
\frac{v_{out}}{v_{sig}} &= \boxed{\left(\frac{-\beta R_{C}}{r_{\pi} + (\beta+1)R_{E}}\right)\left(\frac{R_{in}}{R_{in}+R_{sig}}\right)}\ \ \ \ \ (12)
\end{align}

The last figure we are asked to determine is the output resistance, \(R_{out}\), which is simply:

\begin{equation*}
\boxed{R_{out} = R_{C’}}
\end{equation*}

Remember from Section 2.3 that we find \(R_{out}\) by looking into the terminal where \(R_L \) is connected and as it approaches \(\infty\). We can easily see that \(R_{out} = R_{C’}\) from Figure 11.